Page 22 - WCS - Electrical

3
2
7
0.375 =
8
100
40
273
3

0.375 =
453
=
1000
8
9 375 = 15 15 = 3 3 = (4× 100) + (5× 10) + (3× 1) + 10 2 + 100 7 + 100 3
375
21
7
= 10
= (5×
(4×
=
100) +
0.375 = = = WORKSHOP CALCULATION & SCIENCE - CITS
+
+
+
×
0.875 1)(3) +
16 100 40 3 8 24 8 10 100 100
375
15
7
3
2
0.375 = 3 375 = 15 = 3 = (4× 100) + (5× 10) + (3× 1) + 2 + 7 + 3

273
0.375 =
(4×
+
=
=
100) +
=

0.375 100 375 40 15 8 8 3 = = 453 1000 (5× 10) + (3× 1) + 10 + 2 100 7 100 3
40
100
10
0.5625100
100
= 273
3 8
0.375 =
(5×

1
5
16 0.375 = 3 100 = 40 = 8 = 453 (4× 100) + 625 10) + (3× 1) + 10 + 100 + 100
90000
273
=
=
0.0625 =
0.375 = 8 = 453 1000 10000 80 16
80
1000
9 8 3 = 453 273
0.375 =
100 8 21 7
1000
16
9 9 15 3 2 = 7 = 0.875 2 7 3
96 375
21 84
0.375 = 9 = = = (4× 100) + (5× 10) + = (3× 1) + + +
0.875
=
16
16
40
21
7
100
=
32 9 40 8 24 21 8 8 = 7 0.875 10 100 100

16
0.5625
24
273
3
=

=
5
80 16
0.375 = 2 900 100 = 453 1000 24 625 8 = 0.875 1
1 16
0.5625
0.0625 =
=
, 8
5
625
3 0.5625
1 , 80 0.0625 = 10000 = 80 = 16 1
7
3 900006
1
0 0.5625 10000 80 16
5
625
90000
16
=

9 9 0.0625 = 625 = 5 1
1 10000 9000016 21 7 10000 80 16
= 0.5625
= 9 = 0.3333 − Recurring 0.0625 = = =
=
0.875
=
16 16
3 3 24 8 10000 80 16
9
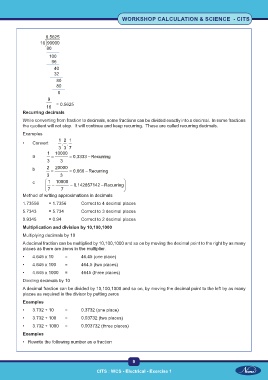
Recurring decimals
16
9
16

While converting from fraction to decimals, some fractions can be divided exactly into a decimal. In some fractions
2
20000 121
0.5625
,
,
=
Recurring
0.666 −
=

16
5
625
1
the quotient will not stop. It will continue and keep recurring. These are called recurring decimals.
16
3 73
90000 121 3
3
0.0625 =
=
=
Examples 1 , , 3 2 , , 7 1 10000 80 16
3
1
1
1
3

7
• Convert 100001 3 10000 2 , 0.3333 − Recurring 
 9 =
, =
3
7
 1 3 = 10000 = 0.14285714 2 − Recurring 
3 3
7
 7 = 10000 = 0.3333 − Recurring 
16
1
a 3 = 3 = 0.3333 − Recurring
3 2 1 20000 =66 − Recurring
10000
3
=
0.3333 −
1
1 2 3 = 2 320000 3 = 0.6 Recurring
3
b , = , = 0.666 − Recurring
2
3 3 20000 = 0.666 − Recurring
7
3
3
=
20000
3
3
c  1 2 10000 = 0.666 − Recurring 
=
1  =  1 7 10000 7 = 3 = 0.14285714 2 − Recurring   
=
3

10000
0.3333 −
Recurring
− Recurring
2
= 0.14285714
3 
=
 1
3 10000
Method of writing approximations in decimals  
− Recurring 
7
 7
2
= 0.14285714


=

 1

= 1.7356
1.73556 2  7 20000 7 10000 = 0.14285714 2 − Recurring 
Correct to 4 decimal places

=


0.666 −
Recurring
5.7343 3 =  7 = 7 Correct to 3 decimal places
= 5.734
3
0.9345 = 0.94 Correct to 2 decimal places

Multiplication and division by 10,100,1000  
 1
10000

2
− Recurring
= 0.14285714
=
Multiplying decimals by 10 
7
 7
A decimal fraction can be multiplied by 10,100,1000 and so on by moving the decimal point to the right by as many
places as there are zeros in the multiplier.
• 4.645 x 10 = 46.45 (one place)
• 4.645 x 100 = 464.5 (two places)
• 4.645 x 1000 = 4645 (three places)
Dividing decimals by 10
A decimal fraction can be divided by 10,100,1000 and so on, by moving the decimal point to the left by as many
places as required in the divisor by putting zeros
Examples
• 3.732 ÷ 10 = 0.3732 (one place)
• 3.732 ÷ 100 = 0.03732 (two places)
• 3.732 ÷ 1000 = 0.003732 (three places)
Examples
• Rewrite the following number as a fraction
9
CITS : WCS - Electrical - Exercise 1 CITS : WCS - Electrical - Exercise 1