Page 14 - CITS - ED - Mechanical

ENGINEERING DRAWING - CITS

EXERCISE 1 : Circles, Tangents and Ellipse

Circles, Tangents and Ellipse

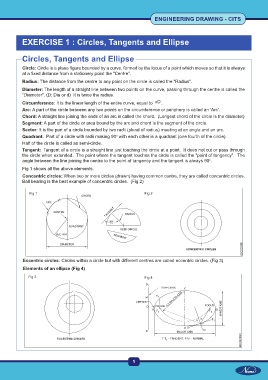
Circle: Circle is a plane figure bounded by a curve, formed by the locus of a point which moves so that it is always
at a fixed distance from a stationery point the "Centre".
Radius: The distance from the centre to any point on the circle is called the "Radius".
Diameter: The length of a straight line between two points on the curve, passing through the centre is called the
"Diameter". (D: Dia or d) It is twice the radius.

Circumference: It is the linear length of the entire curve, equal to .
Arc: A part of the circle between any two points on the circumference or periphery is called an 'Arc'.
Chord: A straight line joining the ends of an arc is called the chord. (Longest chord of the circle is the diameter)
Segment: A part of the circle or area bound by the arc and chord is the segment of the circle.
Sector: It is the part of a circle bounded by two radii (plural of radius) meeting at an angle and an arc.
Quadrant: Part of a circle with radii making 90 with each other is a quadrant (one fourth of the circle).
o
Half of the circle is called as semi-circle.
Tangent: Tangent of a circle is a straight line just touching the circle at a point. It does not cut or pass through
the circle when extended. The point where the tangent touches the circle is called the "point of tangency". The
angle between the line joining the centre to the point of tangency and the tangent is always 90 .
o
Fig 1 shows all the above elements.
Concentric circles: When two or more circles (drawn) having common centre, they are called concentric circles.
Ball bearing is the best example of concentric circles. (Fig 2)

Fig 1 Fig 2

Eccentric circles: Circles within a circle but with different centres are called eccentric circles. (Fig 3)
Elements of an ellipse (Fig 4)

Fig 3 Fig 4

1