Page 99 - Electrician - TT (Volume 2)

ELECTRICIAN - CITS

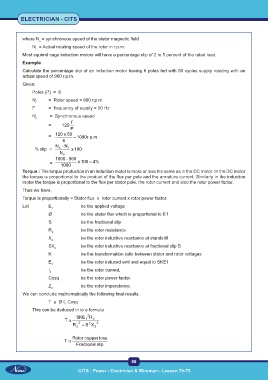
where N = synchronous speed of the stator magnetic field
s
N = Actual rotating speed of the rotor in r.p.m.
r
Most squirrel cage induction motors will have a percentage slip of 2 to 5 percent of the rated load.
Example

Calculate the percentage slip of an induction motor having 6 poles fed with 50 cycles supply rotating with an
actual speed of 960 r.p.m.
Given:

Poles (P) = 6
N = Rotor speed = 960 r.p.m.
r
F = frequency of supply = 50 Hz
N = Synchronous speed
s
= 120 f
P
= 120 x 50 1000r.p.m.
6
N N -
% slip = s r x 100
N s
1000 - 960
= 1000 x 100 4%
Torque : The torque production in an induction motor is more or less the same as in the DC motor. In the DC motor
the torque is proportional to the product of the flux per pole and the armature current. Similarly in the induction
motor the torque is proportional to the flux per stator pole, the rotor current and also the rotor power factor.
Thus we have,
Torque is proportionally = Stator flux x rotor current x rotor power factor.
Let E be the applied voltage
1
Ø be the stator flux which is proportional to E1

S be the fractional slip
R 2 be the rotor resistance
X be the rotor inductive reactance at standstill
2
SX be the rotor inductive reactance at fractional slip S
2
K be the transformation ratio between stator and rotor voltages
E be the rotor induced emf and equal to SKE1
2
I be the rotor current,
2
Cosq be the rotor power factor.
Z be the rotor impendence.
2
We can conclude mathematically the following final results.

T a Ø I Cosq
2
This can be deduced in to a formula
SKE 2 R
T 1 2
R 2 2 S 2 X 2 2

Rotor copper loss
T
Fractional slip

86

CITS : Power - Electrician & Wireman - Lesson 70-75 CITS : Power - Electrician & Wireman - Lesson 70-75