Page 34 - WCS - Electrical

WORKSHOP CALCULATION & SCIENCE - CITS

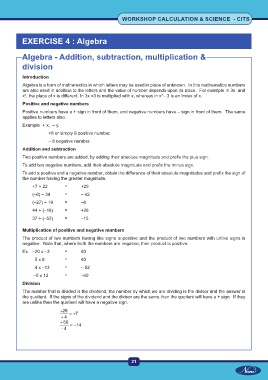
EXERCISE 4 : Algebra

Algebra - Addition, subtraction, multiplication &

division

Introduction

Algebra is a form of mathematics in which letters may be used in place of unknown. In this mathematics numbers
are also used in addition to the letters and the value of number depends upon its place. For example in 3x and
x , the place of x is different. In 3x =3 is multiplied with x, whereas in x - 3 is an Index of x.
3
3
Positive and negative numbers
Positive numbers have a + sign in front of them, and negative numbers have – sign in front of them. The same
applies to letters also.
Example + x, – y.
+8 or simply 8 positive number.
– 8 negative number.
Addition and subtraction

Two positive numbers are added, by adding their absolute magnitude and prefix the plus sign.
To add two negative numbers, add their absolute magnitude and prefix the minus sign.
To add a positive and a negative number, obtain the difference of their absolute magnitudes and prefix the sign of
the number having the greater magnitude.

+7 + 22 = +29
(–8) – 34 = – 42
(–27) + 19 = –8
44 + (–18) = +26

37 + (–52) = –15

Multiplication of positive and negative numbers
The product of two numbers having like signs is positive and the product of two numbers with unlike signs is
negative. Note that, where both the numbers are negative, their product is positive.

Ex. –20 x –3 = 60
5 x 8 = 40
4 x –13 = – 52
–5 x 12 = –60

Division
The number that is divided is the dividend, the number by which we are dividing is the divisor and the answer is
the quotient. If the signs of the dividend and the divisor are the same then the quotient will have a + sign. If they
are unlike then the quotient will have a negative sign.
28+ = + 7
28+
7
+
=
4
+
4
+ + - 56 = − 14
+
56
14
−
=
4
4
72
- - - 72 = −8
+ 9 = −8
9
+
− 96 = +16
− 96
− 6 = +16
− 6
5y = 20
20
5y
=
5
x 5 5 5 21
x
4
4
5x = 25
25
5x
5
5 = 5
5
10a
10a
2a
2a
8ac
− 8ac
−
2bc
− 2bc 6n − 7p
5m −×
5m −×
7p
−
6n −
28mn
−
28mn
5a + − 20
20
5a +
7a + 28
28
7a +
m
am = am− n
m−
a
=
n
a
an
a 3 n
23
3
2 = 3 2 = 1 2
1
2 =
2 −
=
2
2 =
2 −
2 =
22
2 2 2× 2 8
2×
2× 2× 2 = 8 = 2
=
2
=
4
2×
2
2× 2 4
a 
an n =    a   n n
a
=
b 

bn n 3   b   2
b
23
4
2×
2 
2 =     2    2 = 2× 2 = 4
2
=
=
=
2
3 
32   3  3× 3 9
3×
9
3
3
n
1
−
−
a n = 1 n
a
=
an
a 1
2
−
1
2 2
2 − = 22
=
2
2 2 2
   1  2 =    7   2
3 
7 
3 
1 

4 
4 
 4  =    4  

7
7
49
7 × 7 = 49
×
=
4
4
16
4 4 16