Page 29 - CITS - WCS - Mechanical

WORKSHOP CALCULATION - CITS
5 Find out the length of the belt , if the arrangement of a
Area A = x πr unit 2 belt is shown in the figure below.
2
105° 22
= x (4.86) cm 2 = x 2 x x 5 = 91.7 cm
360° 7
= 21.65 cm 2 = l + l + 2 x 214 cm
A
B
4 Find the area, if the radius is 12.4 cm and the perimeter = 110 + 9.17 + 428 cm

of a sector of a circle is 64.8 cm.
Given: = 547.17 cm
Perimeter P = 64.8 cm Hexagon
Radius r = 12.4 cm Solution:
To find:
Length  = x 2πr unit
Area A = ? A
Solution:
210 0

Perimeter P =  + 2r unit = 360 0 x 2 x x 30 = 110 cm
Side = a unit
 = P - 2r unit
Perimeter P = 6a unit
= 64.8 - 2 (12.4) cm Length  = x 2πr unit
B
= 64.8 - 24.8 = 40 cm Area A = 6 x x a² units² (Area of 6 equilateral triangle)
DAF (Distance Across Flats) = x a unit
0
105
lr 40  12.4 = 0 x 2 x x 5 = 91.7 cm

Area A = unit = DAC (Distance Across Corners) = 2 x a unit
360
2
2 2
=  +  + 2 x 214 cm
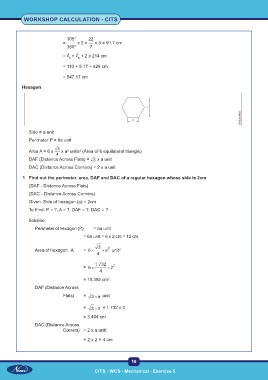
= 248 cm 2 1 Find out the perimeter, area, DAF and DAC of a regular hexagon whose side is 2cm
A
B
(DAF - Distance Across Flats)
= 110 + 9.17 + 428 cm
(DAC - Distance Across Corners)
= 547.17 cm
Given: Side of hexagon (a) = 2cm
Hexagon To Find: P = ?, A = ?, DAF = ?, DAC = ?
Solution:
Perimeter of hexagon (P) = 6a unit
= 6a unit = 6 x 2 cm = 12 cm

3
2
Area of hexagon A = 6  4  a unit 2
Side = a unit
Perimeter P = 6a unit = 6  1.732  2 2
4
3
2
2
Area A = 6   a units (Area of 6 equilateral triangle) = 10.392 cm 2
4
DAF (Distance Across
DAF (Distance Across Flats) = 3  a unit
Flats) = 3  a unit
DAC (Distance Across Corners) = 2 x a unit
1 Find out the perimeter, area, DAF and DAC of a regular = 3  2 = 1.732 x 2
hexagon whose side is 2cm.
= 3.464 cm
(DAF - Distance Across Flats)
DAC (Distance Across
(DAC - Distance Across Corners) Corners) = 2 x a unit
Given: Side of hexagon (a) = 2cm
= 2 x 2 = 4 cm
To Find: P = ?, A = ?, DAF = ?, DAC = ?

st
68 WCS - Electrician & Wireman : (NSQF - Revised 2022) - 1 Year : Exercise 1.7.26
16
CITS : WCS - Mechanical - Exercise 5