Page 34 - CITS - WCS - Mechanical

Workshop Calculation & Science - Electronics Mechanic
Exercise 1.6.25
Workshop Calculation & Science - Electronics Mechanic& Science - Electronics Mechanic
Exercise 1.6.25
Exercise 1.6.25
Workshop Calculation
Trigonometry - Measurement of angles
Trigonometry - Measurement of angles
Trigonometry - Measurement of angles
Workshop Calculation & Science - Electronics Mechanic
Exercise 1.6.25
Introduction:
Introduction:
Introduction:
Circumference makes an angle (2πr) = 360°
Circumference makes an angle (2πr) = 360°
Workshop Calculation & Science - Electronics Mechanic
Exercise 1.6.25
Trigonometry - Measurement of angles
Trigonometry is the branch of mathematics which deals
Trigonometry is the branch of mathematics which deals
Trigonometry is the branch of mathematics which deals
Radius of the circle makes an angle (r) = 1 Radian
Radius of the circle makes an angle (r) = 1 Radian Radius of the circle makes an angle (r) = 1 Radian
with the study of measurement and relationship of the
with the study of measurement and relationship of the
with the study of measurement and relationship of the
Trigonometry - Measurement of angles
o o
Exercise 1.6.25
o
360
C C
three sides and three angles of a triangle.
360
360
three sides and three angles of a triangle.
three sides and three angles of a triangle.
C
Introduction:
Circumference makes an angle (2πr) = 360°
=
ie :
ie :
=
ie :
=
1Radian
r r
1Radian
Units:
1Radian
r
Units:
Trigonometry - Measurement of angles
Units:
Introduction:
Trigonometry is the branch of mathematics which deals
Circumference makes an angle (2πr) = 360°
Radius of the circle makes an angle (r) = 1 Radian
with the study of measurement and relationship of the
Measurement of Angles
o o
Measurement of Angles
Measurement of Angles
o
r r
360
2π
Trigonometry is the branch of mathematics which deals
o
360
2π
r
360
2π
Radius of the circle makes an angle (r) = 1 Radian
360
C
three sides and three angles of a triangle.
Introduction:
with the study of measurement and relationship of the Circumference makes an angle (2πr) = 360°
=
=
=
There are three systems of measuring the angle:
There are three systems of measuring the angle:
There are three systems of measuring the angle:
ie :
=
r r
1Radian
1Radian
1Radian
r
o
360
rC
Units:
three sides and three angles of a triangle.
Trigonometry is the branch of mathematics which deals
Radius of the circle makes an angle (r) = 1 Radian
(i) Sexagesimal System
(i) Sexagesimal System
(i) Sexagesimal System
ie :
with the study of measurement and relationship of the
o o
1Radian
o
r
360
Units:
Measurement of Angles
o
360
360
2π
360
o
r
360
This is called British System. In this system, one right C
three sides and three angles of a triangle.
This is called British System. In this system, one right
This is called British System. In this system, one right
2π =
2π =
2π =
=
1Radian
Measurement of Angles
There are three systems of measuring the angle:
1Radian
ie :
1Radian
=
o
angle is divided into 90 equal parts which are called
1Radian
360
angle is divided into 90 equal parts which are called
angle is divided into 90 equal parts which are called
2π
r r 1Radian
r
Units:
degrees.Each part is divided into 60 parts which are called
=
degrees.Each part is divided into 60 parts which are called
degrees.Each part is divided into 60 parts which are called
2π Radian = 360°
(i) Sexagesimal Systemof measuring the angle:
There are three systems
2π Radian = 360°
2π Radian = 360°
1Radi
r
oan
o
Measurement of Angles minutes.Each minute is divided into 60 parts which are
360
minutes.Each minute is divided into 60 parts which are
minutes.Each minute is divided into 60 parts which are
r
2π
(i) Sexagesimal System
This is called British System. In this system, one right
π Radian = 180°
called seconds.The parts so divided respectively are called:
π Radian = 180°
2π =
π Radian = 180°
called seconds.The parts so divided respectively are called:
called seconds.The parts so divided respectively are called: =
o
There are three systems of measuring the angle:
1Radian
360
1Radian
r
angle is divided into 90 equal parts which are called
This is called British System. In this system, one right
o o
o
One degree (1°), one minute (1') and one second (1")
2π =
180
One degree (1°), one minute (1') and one second (1") One degree (1°), one minute (1') and one second (1")
180
180
degrees.Each part is divided into 60 parts which are called
(i) Sexagesimal System
2π Radian = 360°adian
1R
angle is divided into 90 equal parts which are called
1 Radian =
1 Radian =
1 Radian =
o
360
minutes.Each minute is divided into 60 parts which are
It means 1 right angle
It means 1 right angle = 90° (90 degrees)
It means 1 right angle
= 90° (90 degrees)
π π
= 90° (90 degrees)
π
degrees.Each part is divided into 60 parts which are called
This is called British System. In this system, one right
2π Radian = 360°
π Radian = 180°
2π =
called seconds.The parts so divided respectively are called:
1Radian
1 degree (1°) = 60' (60 minutes)
minutes.Each minute is divided into 60 parts which are
π π
1 degree (1°) = 60' (60 minutes)
π
1 degree (1°) = 60' (60 minutes)
angle is divided into 90 equal parts which are called
1° =
o Radian
π Radian = 180°
o
1° =
o Radian
o Radian
1° =
One degree (1°), one minute (1') and one second (1")
called seconds.The parts so divided respectively are called:
180
degrees.Each part is divided into 60 parts which are called
1 minute (1') = 60" (60 seconds)
180
1 minute (1') = 60" (60 seconds) 2π Radian = 360°
1 minute (1') = 60" (60 seconds)
180
180
1 Radian =
minutes.Each minute is divided into 60 parts which are
Examples
= 90° (90 degrees)
It means 1 right angle
o
One degree (1°), one minute (1') and one second (1")
Examples
Examples
π 180
In Trigonometry, mostly this system is used.
In Trigonometry, mostly this system is used.
In Trigonometry, mostly this system is used.
π Radian = 180°
called seconds.The parts so divided respectively are called:
1 Radian =
1 degree (1°) = 60' (60 minutes)
π
It means 1 right angle
= 90° (90 degrees)
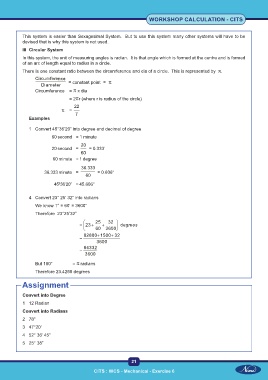
1 Convert 45°36’20” into degree and decimal of degree.
1
1 Convert 45°36’20” into degree and decimal of degree. Convert 45°36’20” into degree and decimal of degree.
π
(ii) Centesimal System
(ii) Centesimal System
(ii) Centesimal System
o
o Radian
1° =
One degree (1°), one minute (1') and one second (1")
180
1 minute (1') = 60" (60 seconds)
1 degree (1°) = 60' (60 minutes)
180 π 60 second = 1 minute
1 Radian =
60 second = 1 minute
60 second = 1 minute
This is called French System. In this system, the right
This is called French System. In this system, the right
This is called French System. In this system, the right
It means 1 right angle
o Radian
1° =
= 90° (90 degrees)
Examples
π
1 minute (1') = 60" (60 seconds)
In Trigonometry, mostly this system is used.
180
angle is divided into 100 equal parts which are called
20
angle is divided into 100 equal parts which are called
angle is divided into 100 equal parts which are called
20
20
1 degree (1°) = 60' (60 minutes)
= 0.333’
20 second =
1 Convert 45°36’20” into degree and decimal of degree.
20 second =
= 0.333’
Examples
= 0.333’
20 second =
grades. Each grade is divided into 100 minutes and each
grades. Each grade is divided into 100 minutes and each
grades. Each grade is divided into 100 minutes and each
(ii) Centesimal System
In Trigonometry, mostly this system is used.
1° =
o Radian
60
60
60
1 minute (1') = 60" (60 seconds)
minute is divided into 100 seconds.
180
minute is divided into 100 seconds.
minute is divided into 100 seconds.
60 second = 1 minute
1 Convert 45°36’20” into degree and decimal of degree.
60 minute = 1 degree
(ii) Centesimal System
60 minute = 1 degree
This is called French System. In this system, the right
60 minute = 1 degree
Examples
Parts so divided are respectively called:
In Trigonometry, mostly this system is used.
Parts so divided are respectively called:
Parts so divided are respectively called:
60 second = 1 minute
angle is divided into 100 equal parts which are called
20
This is called French System. In this system, the right
36.333
= 0.333’
1 Convert 45°36’20” into degree and decimal of degree.
36.333
36.333
20 second =
grades. Each grade is divided into 100 minutes and each
(ii) Centesimal System One grade (1 g), one minute (1' ), one second (1").
One grade (1 g), one minute (1' ), one second (1").
One grade (1 g), one minute (1' ), one second (1").
= 0.606°
36.333 minute =
angle is divided into 100 equal parts which are called
36.333 minute =
36.333 minute =
= 0.606°
= 0.606°
60 20
60
60
minute is divided into 100 seconds.
60
20 second =
60 second = 1 minute
= 0.333’
This is called French System. In this system, the right Exercise 1.6.25
Workshop Calculation & Science - Electronics Mechanic
grades. Each grade is divided into 100 minutes and each
It means 1 right angle = 100 grades (100g)
60 minute = 1 degree
It means 1 right angle = 100 grades (100g)
It means 1 right angle = 100 grades (100g)
60
Parts so divided are respectively called:
minute is divided into 100 seconds.
45
0 36’20” = 45.606°
angle is divided into 100 equal parts which are called
0
20
45 36’20” = 45.606°
45 36’20” = 45.606°
0
1 grade (1 g) = 100 minutes (100’)
1 grade (1 g) = 100 minutes (100’)
1 grade (1 g) = 100 minutes (100’)
60 minute = 1 degree
Trigonometry - Measurement of angles
36.333
20 second =
= 0.333’
grades. Each grade is divided into 100 minutes and each
One grade (1 g), one minute (1' ), one second (1").
Parts so divided are respectively called:
= 0.606°
60
1 minute (1') = 100 seconds (100")
2
36.333 minute = Convert 24.59° into degree, minute and second
2 Convert 24.59° into degree, minute and second
2 Convert 24.59° into degree, minute and second
1 minute (1') = 100 seconds (100")
1 minute (1') = 100 seconds (100")
minute is divided into 100 seconds.
60
36.333
60 minute = 1 degree
It means 1 right angle = 100 grades (100g)
One grade (1 g), one minute (1' ), one second (1").
Introduction: Workshop Calculation & Science - Electronics Mechanic π 360 = 1Radian = 0.606° = 60 minute Circumference makes an angle (2πr) = 360°
36.333 minute =
90° = 100g (because each is a right
90° = 100g (because each is a right 90° = 100g (because each is a right
1 degree
Circumference makes an angle (2πr) = 360°
Parts so divided are respectively called: 45 36’20” = 45.606° 1 degree = 60 minute 1 degree = 60 minute
60
0
angle)
1 grade (1 g) = 100 minutes (100’)
angle)
angle)
It means 1 right angle = 100 grades (100g)
Trigonometry is the branch of mathematics which deals Radius of the circle makes an angle (r) = 1 Radian 36.333 0.59 degree = 0.59 x 60 = 35.4’ 0.59 degree = 0.59 x 60 = 35.4’
0.59 degree = 0.59 x 60 = 35.4’
One grade (1 g), one minute (1' ), one second (1").
36.333 minute =
= 0.606°
45 36’20” = 45.606°
2 Convert 24.59° into degree, minute and second
with the study of measurement and relationship of the This system is easier than Sexagesimal System. But to 0 WORKSHOP CALCULATION - CITS
1 minute (1') = 100 seconds (100")
1 grade (1 g) = 100 minutes (100’)
This system is easier than Sexagesimal System. But to
This system is easier than Sexagesimal System. But to
60
o
1 minute = 60 second
1 minute = 60 second
It means 1 right angle = 100 grades (100g)
C
use this system many other systems will have to be devised
three sides and three angles of a triangle. 1 minute (1') = 100 seconds (100") 360 2 Convert 24.59° into degree, minute and second 1 minute = 60 second
use this system many other systems will have to be devised
use this system many other systems will have to be devised
90° = 100g (because each is a right
1 degree = 60 minute
=
ie :
0
that is why this system is not used.
that is why this system is not used.
that is why this system is not used.
0.4 minute = 60 sec x 0.4
Units: 1 grade (1 g) = 100 minutes (100’) r angle) 1Radian 45 36’20” = 45.606° 0.4 minute = 60 sec x 0.4 0.4 minute = 60 sec x 0.4
90° = 100g (because each is a right
0.59 degree = 0.59 x 60 = 35.4’
1 degree = 60 minute
This system is easier than Sexagesimal System. But to use this system many other systems will have to be
(iii) Circular System
(iii) Circular System
= 24”
This system is easier than Sexagesimal System. But to
(iii) Circular System angle)
Measurement of Angles 1 minute (1') = 100 seconds (100") 2π r 360 o 2 Convert 24.59° into degree, minute and second= 24” = 24”
devised that is why this system is not used.
1 minute = 60 second
0.59 degree = 0.59 x 60 = 35.4’
use this system many other systems will have to be devised
90° = 100g (because each is a right
1 degree
In this system, the unit of measuring angles is radian. It = 60 minute
In this system, the unit of measuring angles is radian. It
=
Therefore 24.59° = 24 35’24”
0
This system is easier than Sexagesimal System. But to
There are three systems of measuring the angle: In this system, the unit of measuring angles is radian. It Therefore 24.59° = 24 0 0 35’24” Therefore 24.59° = 24 35’24”
iii Circular System r
1Radian
0.4 minute = 60 sec x 0.4
that is why this system is not used. angle)formed at the centre and is formed 1 minute = 60 second
is that angle which is
is that angle which is formed at the centre and is formed
is that angle which is formed at the centre and is formed
use this system many other systems will have to be devised
(i) Sexagesimal System of an arc of length equal to radius in a circle. 0.59 degree = 0.59 x 60 = 35.4’ 3 Change 50 37’30” into degrees
3 Change 50
0 37’30” into degrees
0
of an arc of length equal to radius in a circle.
of an arc of length equal to radius in a circle.
3 Change 50 37’30” into degrees
(iii) Circular System
In this system, the unit of measuring angles is radian. It is that angle which is formed at the centre and is formed
0
that is why this system is not used.
= 24”
This system is easier than Sexagesimal System. But to 360 o 0.4 minute = 60 sec x 0.4
1 minute = 60 second
This is called British System. In this system, one right of an arc of length equal to radius in a circle. Therefore 24.59° = 24 35’24” By changing angle degrees into decimals
use this system many other systems will have to be devised
There is one constant ratio between the circumference
By changing angle degrees into decimals
2π =
There is one constant ratio between the circumference
By changing angle degrees into decimals
There is one constant ratio between the circumference
(iii) Circular System
In this system, the unit of measuring angles is radian. It
= 24”
0
1Radian
angle is divided into 90 equal parts which are called There is one constant ratio between the circumference and dia of a circle. This is represented by π . .
that is why this system is not used.
0.4 minute = 60 sec x 0.4
and dia of a circle. This is represented by π .
and dia of a circle. This is represented by
and dia of a circle. This is represented by π .
is that angle which is formed at the centre and is formed
30
In this system, the unit of measuring angles is radian. It
Therefore 24.59° = 24 35’24”
degrees.Each part is divided into 60 parts which are called 2π Radian = 360° 3 Change 50 37’30” into degrees 30” = 30 = 0.50’ 30” = 30 = 0.50’
0
30” =
= 0.50’
of an arc of length equal to radius in a circle.
0
(iii) Circular System
nce
= 24”
60
Circumfere
nce
nce
is that angle which is formed at the centre and is formed
60
minutes.Each minute is divided into 60 parts which are Circumfere = constant point = π Circumfere = constant point = π 60
= constant point = π
of an arc of length equal to radius in a circle. = 180°
π Radian
There is one constant ratio between the circumference
Diameter
Diameter
3 Change 50 37’30” into degrees
By changing angle degrees into decimals
0
called seconds.The parts so divided respectively are called: Diameter Therefore 24.59° = 24 35’24” 37’30” = 37.5’ 37’30” = 37.5’
In this system, the unit of measuring angles is radian. It
0
37’30” = 37.5’
and dia of a circle. This is represented by
is that angle which is formed at the centre and is formed 30
Circumference = π x dia
Circumference = π . x dia o
By changing angle degrees into decimals
There is one constant ratio between the circumference
Circumference
= π x dia
One degree (1°), one minute (1') and one second (1") Circumference = π x dia 3 Change 50 37’30” into degrees = 0.50’ 37.5
180
of an arc of length equal to radius in a circle.
30” =
0
1 Radian =
and dia of a circle. This is represented by
60
= 2πr (where r is radius of the circle)
nce
= 2πr (where r is radius of the circle)
= 2πr (where r is radius of the circle)
37.5’ =
It means 1 right angle = 90° (90 degrees) Circumfere = constant point = π = 2π . r (where r is radius of the circle) 30” = 30 = 0.50’ 37.5’ = 37.5 = 0.625 0 0 37.5’ = 37.5 = 0.625 0
π
= 0.625
60
60
60
There is one constant ratio between the circumference
Diameter
nce
60
22
37’30” = 37.5’
1 degree (1°) = 60' (60 minutes) Circumfere = constant point = π 22 By changing angle degrees into decimals 0
22 π
and dia of a circle. This is represented by π
π . =
π
π =
=
0 37’30” = 50.625
= 0
Diameter
50 37’30” = 50.625
0
7 7
1 minute (1') = 60" (60 seconds) Circumference = π x dia 1° = 180 o Radian 30” = 30 = 0.50’ 50 7 0 50 37’30” = 50.625 0
37’30” = 37.5’
37.5
nce
Circumfere
Examples
60
= 2πr (where r is radius of the circle)
= π x dia
37.5’ =
= constant point = π
59
In Trigonometry, mostly this system is used. Circumference Examples 37’30” = 37.5’ 60 = 0.625 0 59 59
Diameter
37.5
= 2π
22r (where r is radius of the circle)
1 Convert 45°36’20” into degree and decimal of degree.
37.5’ =
1 Convert 45°36’20” into degree and decimal of degree
π
=
(ii) Centesimal System Circumference = π x dia 7 22 50 37’30” = 50.625 = 0.625 0
60
0
0
= 2πr (where r is radius of the circle)
This is called French System. In this system, the right π = 60 second = 1 minute 37.5’ = 37.5 = 0.625 0 0 59
50 37’30” = 50.625
0
angle is divided into 100 equal parts which are called 22 7 20 60
π
grades. Each grade is divided into 100 minutes and each 20 second = 60 = 0.333’ 50 37’30” = 50.625 0 59
=
0
minute is divided into 100 seconds. 7
60 minute = 1 degree
Parts so divided are respectively called: 59
36.333
One grade (1 g), one minute (1' ), one second (1"). 36.333 minute = = 0.606°
It means 1 right angle = 100 grades (100g) 60
0
1 grade (1 g) = 100 minutes (100’) 45 36’20” = 45.606°
1 minute (1') = 100 seconds (100") 2 Convert 24.59° into degree, minute and second
4 Convert 23 25’ 32” into radians
4 Convert 23° 25’ 32” into radians
0
0
90° = 100g (because each is a right 4 Convert 23 25’ 32” into radians 4 4 π radian = 180 x 4 π degree
1 degree = 60 minute
4
180
We know 1 = 60’ = 3600”
We know 1° = 60’ = 3600”
0
angle) We know 1 = 60’ = 3600” 7 π radian = π x π 7 π degree
7
7
0
0.59 degree = 0.59 x 60 = 35.4’
Therefore 23 25’32”
0
Therefore 23°25’32”
= 102.9 degree
This system is easier than Sexagesimal System. But to Therefore 23 25’32” = 102.9 degree
0
use this system many other systems will have to be devised 1 minute = 60 second 32 ⎞ = 102 0.9 x 60’
= 102 0.9 x 60’
0
0
⎛
25
25
32 ⎞
⎛
degrees
=

that is why this system is not used. 0.4 minute = 60 sec x 0.4 ⎟ degrees = 102 54’ 0
+
+
23
⎜
⎟
23

⎜
+
+
=
= 102 54’
60
0
3600 ⎠
⎝ 60
3600 ⎠
⎝
(iii) Circular System = 24” 1500+ 32 32 8 Convert 0.8357 radian into degrees
8 Convert 0.8357 radian into degrees
1500+
82800+
82800+
=
=
In this system, the unit of measuring angles is radian. It Therefore 24.59° = 24 35’24” 3600 180
0
3600
1 radian =
degree
is that angle which is formed at the centre and is formed 84332 1 radian = 180 degree
84332
π
=
of an arc of length equal to radius in a circle. 3 Change 50 37’30” into degrees π
=
0
3600
3600
There is one constant ratio between the circumference By changing angle degrees into decimals 180 180
But 180
= π radians
0
0.8357 radian = π x 0.8357 degree
But 180° = = π radians
But 180
radians
0
and dia of a circle. This is represented by π . Therefore 23.4255 degrees 0.8357 radian = π x 0.8357 degree
30
30” =
= 47.88
Therefore 23.4255 degrees
Circumfere Therefore 23.4255 degrees 60 = 0.50’ = 47.88 0 0
nce
23.4255
= 47 0.88 x 60’
0
23.4255
0
Diameter = constant point = π 37’30” = 37.5’ 180 π radians = 47 0.88 x 60’
radians
=
π
=
= 47 52.80’
0
180
0
Circumference = π x dia Assignment 23.4255 22 22 = 47 52.80’
23.4255
= 47 52’0.8 x 60”
0

radians
=
0
x
= 2πr (where r is radius of the circle) Convert into Degree = 37.5 180 7 x 0 radians = 47 52’0.8 x 60”
= 0.625 7
37.5’ =
180
= 47 52’48”
0
60
0
22 1 12 Radian = 0.4089 radians = 47 52’48”
π = 0 = 0.4089 radians 9 Convert 2.752 radian into degrees
9 Convert 2.752 radian into degrees
50 37’30” = 50.625
0
7 Convert into Radians 180
5 Convert 87 19’ 57” into Radian.
0
5 Convert 87 19’ 57” into Radian.
0
1 Radian =
degree
2 78° 5 7′ 5 7′ 59 1 Radian = 180 degree
π
19’57” = 19’ +
19’57” = 19’ + 60 π
3 47°20’ 60 180 180
= 19’ + 0.95’
2.7520 radian =
x 2.752 degree
π
4 52° 36’ 45” = 19’ + 0.95’ 2.7520 radian = π x 2.752 degree
= 19.95’
= 19.95’ 0
= 157.7
5 25° 38” o = 157.7 0 0
19.95
= 157.7 x 60’
0
87°19.95’ = 87° +
87°19.95’ = 87° + 19.95 o = 157.7 x 60’
= 157 42’
60 60 = 157 42’ 0
0
= 87° + 0.332° = 87.33°
= 87° + 0.332° = 87.33° 21 10 Convent 3 3 π radian into degrees
10 Convent π radian into degrees
5
π
CITS : WCS - Mechanical - Exercise 6
1° =
radian
1° = π 180 radian 5
180 1 Radian = 180 180 degree
1 Radian =
degree
π
87.33° =
x 87.33 radian
87.33° = π 180 x 87.33 radian π π
180 3 3 π radian = 180 3 3 π degree
180
= 1.524 radian
5
5
π
= 1.524 radian 5 π radian = π x 5 x π degree
6 Convert 67°11’43” into Radian
= 108
6 Convert 67°11’43” into Radian = 108 0 0
3′
11’43” = 11’ +
Assignment
11’43” = 11’ + 4 3′ 4 60 Assignment
60
Convert into Degree
= 11’ + 0.716’
= 11’ + 0.716’ Convert into Degree
= 11.72’
1 12 Radian
= 11.72’ o 1 12 Radian
Convert into Radians
11.72
67°11.72’ = 67° +
67°11.72’ = 67° + 11.72 o Convert into Radians
60 60 2 78 o o
2 78
= 67° + 0.195°
= 67° + 0.195° 3 47 20' 0
3 47 20'
0
= 67.2°
= 67.2° 4 52 36' 45"
4 52 36' 45"
0
0
π π 5 25 38"
0
1° =
0
1° = 180 radian 5 25 38"
radian
180 Convert into degree, minute and seconds
Convert into degree, minute and seconds
π
π
67.2° =
6 46.723
x 67.2 radian
67.2° = 180 180 x 67.2 radian 6 46.723 o o
7 68.625
= 1.173 radian
= 1.173 radian 7 68.625 o o
4
4
8 0.1269 Radians
7 Convert π

radian into degrees
7 Convert π 7 radian into degrees 8 0.1269 Radians
7 9 2.625 Radians
9 2.625 Radians
180
180
degree
1 radian =
10 3/5 Radians
1 radian = π degree 10 3/5 Radians
π
WCS - Electronics Mechanic : (NSQF - Revised Syllabus 2022) - 1 Year : Exercise 1.6.25
st
60 60 WCS - Electronics Mechanic : (NSQF - Revised Syllabus 2022) - 1 Year : Exercise 1.6.25
st