Page 117 - WCS - Electrical

WORKSHOP CALCULATION & SCIENCE - CITS

Solution: Mean: Sum of all values = 12 + 15 + 18 + 20 + 22 + 25 + 28 + 30 = 170

Number of values= 8
Mean = Sum of all values / Number of values = 170 / 8 = 21.25
Median: Since the num.ber of observations is even, the median is the average of the two middle values:
Median = (20 + 22) / 2 = 21
Mode: The mode is the value that appears most frequently in the dataset. In this case, there is no mode as all
values occur only once.
Relationship: In this dataset, Mean= 21.25, Median = 21, and there is no mode. The relationship between Mean,
Median, and Mode does not hold in this case due to the absence of repeated values.
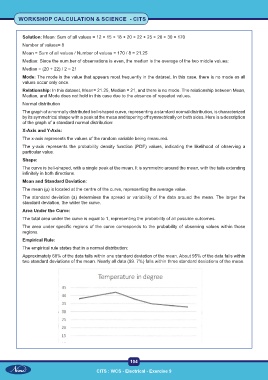
Normal distribution
The graph of a normally distributed bell-shaped curve, representing a standard normal distribution, is characterized
by its symmetrical shape with a peak at the mean and tapering off symmetrically on both sides. Here is a description
of the graph of a standard normal distribution:
X-Axis and Y-Axis:

The x-axis represents the values of the random variable being measured.
The y-axis represents the probability density function (PDF) values, indicating the likelihood of observing a
particular value.
Shape:

The curve is bell-shaped, with a single peak at the mean. It is symmetric around the mean, with the tails extending
infinitely in both directions.
Mean and Standard Deviation:

The mean (μ) is located at the centre of the curve, representing the average value.
The standard deviation (a) determines the spread or variability of the data around the mean. The larger the
standard deviation, the wider the curve.
Area Under the Curve:

The total area under the curve is equal to 1, representing the probability of all possible outcomes.
The area under specific regions of the curve corresponds to the probability of observing values within those
regions.

Empirical Rule:
The empirical rule states that in a normal distribution:
Approximately 68% of the data falls within one standard deviation of the mean. About 95% of the data falls within
two standard deviations of the mean. Nearly all data (99. 7%) falls within three standard deviations of the mean.

104

CITS : WCS - Electrical - Exercise 9