Page 91 - WCS - Electrical

Workshop Calculation & Science - Electronics Mechanic Exercise 1.6.25
WORKSHOP CALCULATION & SCIENCE - CITS
Trigonometry - Measurement of angles

Workshop Calculation & Science - Electronics Mechanic Exercise 1.6.25
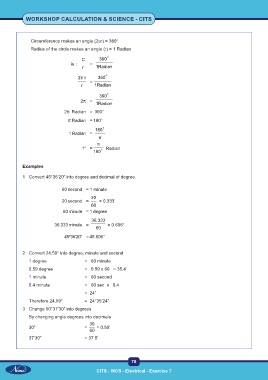
Workshop Circumference makes an angle (2pr) = 360°
Exercise 1.6.25
Introduction:Calculation & Science - Electronics Mechanic
Circumference makes an angle (2πr) = 360°
Trigonometry - Measurement of angles
Trigonometry is the branch of mathematics which deals
Radius of the circle makes an angle (r) = 1 Radian
Trigonometry - Measurement of angles Radius of the circle makes an angle (r) = 1 Radian
with the study of measurement and relationship of the
Introduction: Circumference makes an angle o
360(2πr) = 360°
three sides and three angles of a triangle.
C
Introduction: Circumference makes an angle (2πr) = 360°
ie :
=
Trigonometry is the branch of mathematics which deals Radius of the circle makes an angle (r) = 1 Radian
1Radian
r
Units:
Trigonometry is the branch of mathematics which deals Radius of the circle makes an angle (r) = 1 Radian
with the study of measurement and relationship of the
with the study of measurement and relationship of the C r 360 o o
Measurement of Angles
three sides and three angles of a triangle.
2π
360 o
360
three sides and three angles of a triangle. ie : C = = 1Radian
There are three systems of measuring the angle:
Units: ie : r r = 1Radian
Units: r 1Radian
(i) Sexagesimal System
Measurement of Angles 2π r 360 o o
Measurement of Angles 2π r 360 360
o
=
This is called British System. In this system, one right
There are three systems of measuring the angle: r 2π = 1Radian
1Radian
There are three systems of measuring the angle: r = 1Radian
angle is divided into 90 equal parts which are called
(i) Sexagesimal System
degrees.Each part is divided into 60 parts which are called
360
(i) Sexagesimal System 2π Radian = 360° o
minutes.Each minute is divided into 60 parts which are
This is called British System. In this system, one right 2π = 360 o
1Radian
This is called British System. In this system, one right π Radian = 180°
called seconds.The parts so divided respectively are called:
angle is divided into 90 equal parts which are called
2π =
1Radian
angle is divided into 90 equal parts which are called 2π Radian = 360° o
degrees.Each part is divided into 60 parts which are called
One degree (1°), one minute (1') and one second (1")
180
degrees.Each part is divided into 60 parts which are called 2π Radian = 360°
minutes.Each minute is divided into 60 parts which are
1 Radian =
minutes.Each minute is = 90° (90 degrees) π Radian = 180° π
It means 1 right angledivided into 60 parts which are
called seconds.The parts so divided respectively are called:
called seconds.The parts so divided respectively are called: π Radian = 180°
1 degree (1°) = 60' (60 minutes)
π o
One degree (1°), one minute (1') and one second (1") 180
1° = o
One degree (1°), one minute (1') and one second (1") 1 Radian = 180 o Radian
180
1 minute (1') = 60" (60 seconds)
= 90° (90 degrees)
It means 1 right angle
π
It means 1 right angle = 90° (90 degrees) Examples 1 Radian = π
In Trigonometry, mostly this system is used.
1 degree (1°) = 60' (60 minutes)
π
1° =
1 degree (1°) = 60' (60 minutes) Examples 1 Convert 45°36’20” into degree and decimal of degree.
π o Radian
(ii) Centesimal System
1 minute (1') = 60" (60 seconds)
180
1° =
o Radian
1 minute (1') = 60" (60 seconds) Examples 60 second = 1 minute
180
This is called French System. In this system, the right
In Trigonometry, mostly this system is used. 1 Convert 45°36’20” into degree and decimal of degree.
Examples
In Trigonometry, mostly this system is used. 1 Convert 45°36’20” into degree and decimal of degree.
angle is divided into 100 equal parts which are called
20
(ii) Centesimal System 20 second = = 0.333’
grades. Each grade is divided into 100 minutes and each 1 Convert 45°36’20” into degree and decimal of degree.
(ii) Centesimal System 60 second = 1 minute
60
minute is divided into 100 seconds.
This is called French System. In this system, the right 60 second = 1 minute
This is called French System. In this system, the right 60 minute = 1 degree
angle is divided into 100 equal parts which are called
20
Parts so divided are respectively called:
angle is divided into 100 equal parts which are called 20 second = 20 = 0.333’
grades. Each grade is divided into 100 minutes and each
36.333
20 second = 60
grades. Each grade is divided into 100 minutes and each 36.333 minute = = 0.333’
One grade (1 g), one minute (1' ), one second (1").
minute is divided into 100 seconds.
= 0.606°
60
60e
minute is divided into 100 seconds. 60 minute = 1 degre
It means 1 right angle = 100 grades (100g)
Parts so divided are respectively called: 60 minute = 1 degree
Parts so divided are respectively called: 45 36’20” = 45.606°
36.333
0
1 grade (1 g) = 100 minutes (100’)
One grade (1 g), one minute (1' ), one second (1"). 36.333 minute = 36.333 = 0.606°
One grade (1 g), one minute (1' ), one second (1"). 2 Convert 24.59° into degree, minute and second
60
36.333 minute =
= 0.606°
1 minute (1') = 100 seconds (100")
It means 1 right angle = 100 grades (100g)
60
It means 1 right angle = 100 grades (100g) 45 36’20” = 45.606°
90° = 100g (because each is a right
0
1 degree = 60 minute
1 grade (1 g) = 100 minutes (100’)
45 36’20” = 45.606°
0
1 grade (1 g) = 100 minutes (100’) angle) 2 Convert 24.59° into degree, minute and second
1 minute (1') = 100 seconds (100")
0.59 degree = 0.59 x 60 = 35.4’
This system is easier than Sexagesimal System. But to 2 Convert 24.59° into degree, minute and second
1 minute (1') = 100 seconds (100") 2 Convert 24.59° into degree, minute and second
1 minute = 60 second
90° = 100g (because each is a right
1 degree = 60 minute
use this system many other systems will have to be devised
90° = 100g (because each is a right 1 degree = 60 minute
angle)
= 60 minute
that is why this system is not used. angle) 1 degree 0.59 degree = 0.59 x 60 = 35.4’
0.4 minute = 60 sec x 0.4
This system is easier than Sexagesimal System. But to 0.59 degree = 0.59 x 60 = 35.4’
(iii) Circular System
= 0.59 x 60 = 35.4’

= 24”
This system is easier than Sexagesimal System. But to 0.59 degree 1 minute = 60 second
use this system many other systems will have to be devised
1 minute = 60 second
use this system many other systems will have to be devised 1 minute Therefore 24.59° = 24 35’24”

= 60 second
In this system, the unit of measuring angles is radian. It
that is why this system is not used.
0
0.4 minute = 60 sec x 0.4
that is why this system is not used. 0.4 minute = 60 sec x 0.4
is that angle which is formed at the centre and is formed
(iii) Circular System 0.4 minute = 60 sec x 0.4
= 24”
of an arc of length equal to radius in a circle.
0
(iii) Circular System 3 Change 50 37’30” into degrees
= 24”

In this system, the unit of measuring angles is radian. It Therefore 24.59° = 24 35’24”
= 24” 0
There is one constant ratio between the circumference
By changing angle degrees into decimals
In this system, the unit of measuring angles is radian. It Therefore 24.59° = 24 35’24”
0
is that angle which is formed at the centre and is formed

and dia of a circle. This is represented by π .
30
is that angle which is formed at the centre and is formed Therefore 24.59° 0 = 24°35’24”
3 Change 50 37’30” into degrees
of an arc of length equal to radius in a circle.
30” =
of an arc of length equal to radius in a circle. 3 Change 50°37’30” into degrees 60 = 0.50’
3 Change 50 37’30” into degrees
Circumfere
0
nce
There is one constant ratio between the circumference By changing angle degrees into decimals
= constant point = π
Diameter
There is one constant ratio between the circumference By changing angle degrees into decimals
By changing angle degrees into decimals
and dia of a circle. This is represented by π .
37’30” = 37.5’
30
and dia of a circle. This is represented by π . 30” = 30 = 0.50’
= π x dia
Circumference
Circumfere 30” 30” = = 60 = 0.50’
nce
= 0.50’
nce
Circumfere = constant point = π 37.5’ = 37.5 = 0.625 0
= 2πr (where r is radius of the circle)
60
Diameter
60
Diameter = constant point = π 37’30” 37’30” = 37.5’
22
= 37.5’
Circumference π = π x dia 37’30” = 37.5’
=
0
Circumference = π x dia 50 37’30” = 50.625 0
37.5
7
= 2πr (where r is radius of the circle)
37.5’ =
= 2πr (where r is radius of the circle) 37.5’ = 37.5 = 0.625 0 59
60
= 0.625
0
22
60
π = 22 50 37’30” = 50.625 0
0
π = 7 50 37’30” = 50.625 0 78
0
7 59
CITS : WCS - Electrical - Exercise 7
59