Page 64 - CITS - WCS - Mechanical

WORKSHOP SCIENCE - CITS

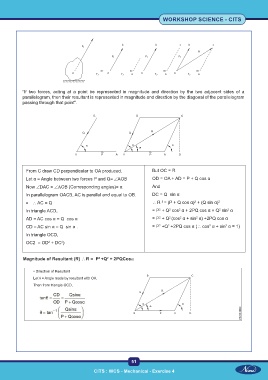
"If two forces, acting at a point be represented in magnitude and direction by the two adjacent sides of a
parallelogram, then their resultant is represented in magnitude and direction by the diagonal of the parallelogram
passing through that point".

From C draw CD perpendicular to OA produced. But OC = R

Let α = Angle between two forces P and Q= ∠AOB OD = OA + AD = P + Q cos α
Now ∠DAC = ∠AOB (Corresponding angles)= α And
In parallelogram OACB, AC is parallel and equal to OB. DC = Q sin α
2
• \ AC = Q \ R = (P + Q cos α) + (Q sin α) 2
2
2
2
2
2
2
In triangle ACD, = P + Q cos α + 2PQ cos α + Q sin α
2
AD = AC cos α = Q cos α = P + Q (cos α + sin α) +2PQ cos α
2
2
2
2
2
2
2
CD = AC sin α = Q sin α . = P +Q +2PQ cos α (\ cos α + sin α = 1)
In triangle OCD,
OC2 = OD + DC )
2
2
Magnitude of Resultant (R) \R = P +Q + 2PQCosa
2
2
• Direction of Resultant
Let q = Angle made by resultant with OA.
Then from triangle OCD,

51

CITS : WCS - Mechanical - Exercise 4 CITS : WCS - Mechanical - Exercise 4