Page 29 - CITS - ED - Mechanical

ENGINEERING DRAWING - CITS

Note: Rectangular hyperbola is a graphical representation of Boyle's law, PV = constant. This curve
also finds application in the design of water channels.

Exercise 2.8
Construct a hyperbola passing through a given point between the asymptotes making any angle other than 90°.
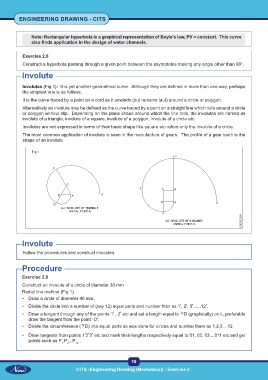
Involute

Involutes (Fig 1): It is yet another geometrical curve. Although they are defined in more than one way, perhaps
the simplest one is as follows.
It is the curve traced by a point on a cord as it unwinds (but remains taut) around a circle or polygon.
Alternatively an involute may be defined as the curve traced by a point on a straight line which rolls around a circle
or polygon without slip. Depending on the plane shape around which the line rolls, the involutes are named as
involute of a triangle, involute of a square, involute of a polygon, involute of a circle etc.
Involutes are not expressed in terms of their basic shape like square etc refers only the involute of a circle.
The most common application of involute is seen in the manufacture of gears. The profile of a gear tooth is the
shape of an involute.

Fig 1

Involute

Follow the procedures and construct involutes.

Procedure

Exercise 2.9
Construct an involute of a circle of diameter 30 mm
Radial line method (Fig 1)
• Draw a circle of diameter 40 mm.
• Divide the circle into a number of (say 12) equal parts and number than as 1', 2', 3'......12'.
• Draw a tangent through any of the points 1' , 2' etc and set a length equal to D (graphically) on it, preferable
draw the tangent from the point `O'.
• Divide the circumference ( D) into equal parts as was done for circles and number them as 1,2,3....12.
• Draw tangents from points 1'2'3' etc and mark their lengths respectively equal to 01, 02, 03....011 etc and get
points such as P ,P ...P .
12
2
1
16
CITS :Engineering Drawing (Mechanical) - Exercise 2