Page 26 - CITS - ED - Mechanical

ENGINEERING DRAWING - CITS

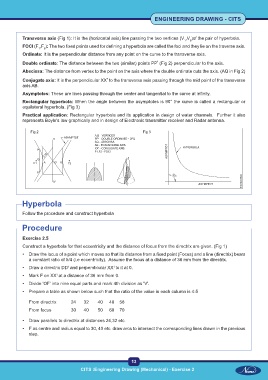
Transverse axis (Fig 1): It is the (horizontal axis) line passing the two vertices (V ,V )of the pair of hyperbola.
2
1
FOCI (F ,F ): The two fixed points used for defining a hyperbola are called the foci and they lie on the traverse axis.
1
2
Ordinate: It is the perpendicular distance from any point on the curve to the transverse axis.
Double ordinate: The distance between the two (similar) points PP' (Fig 2) perpendicular to the axis.
Abscissa: The distance from vertex to the point on the axis where the double ordinate cuts the axis. (AQ in Fig 2)
Conjugate axis: It is the perpendicular XX' to the transverse axis passing through the mid point of the transverse
axis AB.
Asymptotes: These are lines passing through the center and tangential to the curve at infinity.
Rectangular hyperbola: When the angle between the asymptotes is 90° the curve is called a rectangular or
equilateral hyperbola. (Fig 3)
Practical application: Rectangular hyperbola and its application in design of water channels. Further it also
represents Boyle's law graphically and in design of Electronic transmitter receiver and Radar antenna.

Fig 2 Fig 3

Hyperbola

Follow the procedure and construct hyperbola

Procedure

Exercise 2.5
Construct a hyperbola for that eccentricity and the distance of focus from the directrix are given. (Fig 1)

• Draw the locus of a point which moves so that its distance from a fixed point (Focus) and a line (directrix) bears
a constant ratio of 5/4 (i.e eccentricity). Assume the focus at a distance of 36 mm from the directrix.
• Draw a directrix DD and perpendicular XX to it at 0.
1
1
• Mark F on XX at a distance of 36 mm from 0.
1
• Divide 'OF' into nine equal parts and mark 4th division as 'V'.
• Prepare a table as shown below such that the ratio of the value in each column is 4:5

From directrix 24 32 40 48 56
From focus 30 40 50 60 70

• Draw parallels to directrix at distances 24,32 etc.
• F as centre and radius equal to 30, 40 etc. draw arcs to intersect the corresponding lines drawn in the previous
step.

13
CITS :Engineering Drawing (Mechanical) - Exercise 2