Page 22 - CITS - ED - Mechanical

ENGINEERING DRAWING - CITS

EXERCISE 2 :Parabolic curves, Hyperbola, Involute and

Helix

Parabolic curves, Hyperbola, Involute and Helix

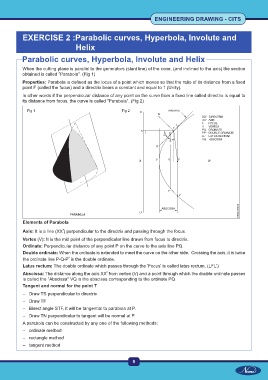
When the cutting plane is parallel to the generators (slant line) of the cone, (and inclined to the axis) the section
obtained is called "Parabola". (Fig 1)
Properties: Parabola is defined as the locus of a point which moves so that the ratio of its distance from a fixed
point F (called the focus) and a directrix bears a constant and equal to 1 (Unity).

In other words if the perpendicular distance of any point on the curve from a fixed line called directrix is equal to
its distance from focus, the curve is called "Parabola". (Fig 2)

Fig 1 Fig 2

Elements of Parabola
Axis: It is a line (XX') perpendicular to the directrix and passing through the focus.
Vertex (V): It is the mid point of the perpendicular line drawn from focus to directrix.
Ordinate: Perpendicular distance of any point P on the curve to the axis line PQ.
Double ordinate: When the ordinate is extended to meet the curve on the other side. Crossing the axis, it is twice
the ordinate line P-Q-P' is the double ordinate.
Latus rectum: The double ordinate which passes through the 'Focus' is called latus rectum. (LFL')
Abscissa: The distance along the axis XX' from vertex (V) and a point through which the double ordinate passes
is called the "Abscissa" VQ is the abscissa corresponding to the ordinate PQ.
Tangent and normal for the point T
– Draw TS perpendicular to directrix
– Draw TF
– Bisect angle STF, it will be tangential to parabola at P.
– Draw TN perpendicular to tangent will be normal at P.

A parabola can be constructed by any one of the following methods:
– ordinate method
– rectangle method
– tangent method

9