Page 66 - WCS - Electrical

h
19.44
5.4
=
h = 19.44 x 2 x 2
= 7.2 m 5.4
= 7.2 m
13 Calculate the base of a right angled triangle having an WORKSHOP CALCULATION & SCIENCE - CITS
area of 15 cm . If its height is 3.5 cm.
2
13 Calculate the base of a right angled triangle having an
area of 15 cm . If its height is 3.5 cm.
2
1 x base x height unit 2 = Area
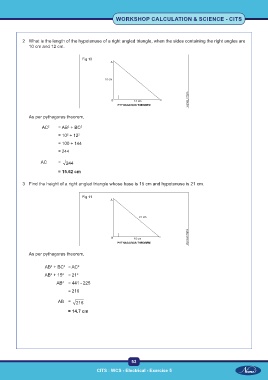
2 1 x base x height unit 2 = Area 2 What is the length of the hypotenuse of a right angled triangle, when the sides containing the right angles are
2 What is the length of the hypotenuse of a right angled
2 1 10 cm and 12 cm.
triangle, when the sides containing the right angles
x b x 3.5 = 15 2 What is the length of the hypotenuse of a right angled
triangle, when the sides containing the right angles
2 1 x b x 3.5 = 15 are 10 cm and 12 cm.
2 x 15 2 are 10 cm and 12 cm.
b = x 15 2
3.5
b =
3.5
= 8.57 cm
= 8.57 cm
Pythagoras theorem
Pythagoras theorem
In a right angled triangle the area of the square drawn with
the hypotenuse as the side is equal to the sum of the
In a right angled triangle the area of the square drawn with
areas of the squares drawn with the other two sides.
the hypotenuse as the side is equal to the sum of the
areas of the squares drawn with the other two sides. As per pythagoras theorem,
As per pythagoras theorem,
As per pythagoras theorem,
AC
= AB + BC
2
2
2
= AB + BC
AC 2 = 10 + 12 2 2
2
2
= 10 + 12
2
= 100 + 144 2
= 100 + 144
= 244
= 244
AC = 244
AC = 244
= 15.62 cm
= 15.62 cm
3 Find the height of a right angled triangle whose base is
B∠ = 90º 3 Find the height of a right angled triangle whose base is 15 cm and hypotenuse is 21 cm.
15 cm and hypotenuse is 21 cm.
3 Find the height of a right angled triangle whose base is
= 90º
AC B∠ = Hypotenuse 15 cm and hypotenuse is 21 cm.
= Hypotenuse
AC
AB & BC = Adjacent sides
AB & BC = Adjacent sides
As per pythagoras theorem,
As per pythagoras theorem,
= AB + BC
AC
2
2
2
AC 2 = AB + BC 2
2

2
∴ AC = AB + BC 2

2
∴ AC = AB + BC 2
1 Calculate the hypotenuse of a right angled triangle
whose base is 5 cm and height is 12 cm.
1 Calculate the hypotenuse of a right angled triangle
whose base is 5 cm and height is 12 cm.
As per pythagoras theorem, As per pythagoras theorem,
As per pythagoras theorem,
As per pythagoras theorem, AB + BC = AC 2
AC
As per pythagoras theorem,
= AB + BC
2
2
2
2
2
= AB + BC
AC 2 = 12 + 5 2 2 2 AB + 15 2 = 21 2 2
AB + BC = AC
2
2
2
2
= 12 + 5
2
= 21
= 144 + 25 2 AB + 15 = 441 - 225
2
2
2
AB
2
= 144 + 25
= 441 - 225
= 169 AB = 216
2
= 169 = 216
AC = 169 AB = 216
AC = 169 AB =
216
= 13 cm = 14.7 cm
= 13 cm = 14.7 cm
WCS - Electrician & Wireman : (NSQF - Revised 2022) - 1 Year : Exercise 1.7.25 63
st
WCS - Electrician & Wireman : (NSQF - Revised 2022) - 1 Year : Exercise 1.7.25 63
st
53
CITS : WCS - Electrical - Exercise 5