Page 68 - WCS - Electrical

Exercise 1.7.26
Workshop Calculation & Science - Electrician & Wireman
Mensuration - Area and perimeter of circle, semi-circle, circular ring, sector
of circle, hexagon and ellipse
Circle
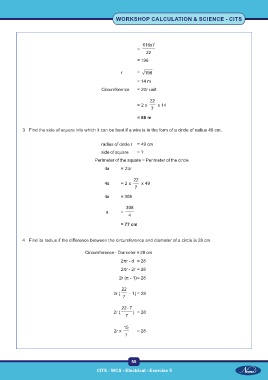
r
= 196
It is the path of a point which is always equal from its
centre is called a circle.
= 14 m
r = radius of the circle
Circumference
= 2πr unit
d = diameter of the circle
22
22
x 14
= 2 x
π =
= 3.14
7
7
= 88 m
Area of the circle = πr
2
3 Find the side of square into which it can be bent if a
wire is in the form of a circle of radius 49 cm.
= 49 cm
radius of circle r
side of square
= ?
Perimeter of the square = Perimeter of the circle
4a
= 2πr
22
π
= 2 x
x 49
4a

(or)
7
d unit
=
2
2
4
= 308
4a
Circumference of the circle 2πr (or) πd unit
Examples
308
a
=
1 Find the area of a circle whose radius is 1.54 m. Also
4
find its circumference.
= 77 cm
radius r
= 1.54 cm
4 Find its radius if the difference between the
Area A
= ?
circumference and diameter of a circle is 28 cm.
= ?
Circumference C
Circumference - Diameter = 28 cm
A
= πr unit
2
2
2πr - d = 28
22
x 1.54 x 1.54
=
2πr - 2r = 28
7
2r (π - 1)= 28
= 7.4536 m
2
= 2πr unit
C
2r (
22
7
= 2 x
x 1.54
7
7
22
= 9.68 m
2r ( 22 - - 1) = 28
) = 28
2 Find out the circumference if the area of a circular shape 7
of land is 616 m .
2
WORKSHOP CALCULATION & SCIENCE - CITS 2r x 15 = 28
= πr unit
A
2
2

616 7
=
r
2

Workshop Calculation & Science - Electrician & Wireman π Exercise 1.7.26 28x7
Workshop Calculation & Science - Electrician & Wireman Exercise 1.7.26 r =
616x7
Workshop Calculation & Science - Electrician & Wireman Exercise 1.7.26 15x2
Mensuration - Area and perimeter of circle, semi-circle, circular ring, sector
=
Mensuration - Area and perimeter of circle, semi-circle, circular ring, sector
22
of circle, hexagon and ellipse
Mensuration - Area and perimeter of circle, semi-circle, circular ring, sector = 6.53 cm
of circle, hexagon and ellipse
of circle, hexagon and ellipse = 196
Circle

Circle 64 r = 196

Circle It is the path of a point which is always equal from its r = 196

centre is called a circle.
It is the path of a point which is always equal from its r = 196 = 14 m
It is the path of a point which is always equal from its = 14 m
centre is called a circle.
centre is called a circle. r = radius of the circle Circumference = 2πr unit
= 14 m
r = radius of the circle Circumference = 2πr unit
d = diameter of the circle
r = radius of the circle Circumference = 2πr unit
d = diameter of the circle 22
22
= 2 x
d = diameter of the circle 22 = 2 x 22 x 14
= 3.14
7
π =
22
22 π = 7 = 3.14 = 2 x x 14 7 x 14
π = = 3.14 7 7= 88 m
Area of the circle = πr 2 = 88 m
7
Area of the circle = πr 2 3 Find the side of square into which it can be bent if a
= 88 m
Area of the circle = πr 2 3 Find the side of square into which it can be bent if a wire is in the form of a circle of radius 49 cm.
3 Find the side of square into which it can be bent if a
wire is in the form of a circle of radius 49 cm.
3 Find the side of square into which it can be bent if a
wire is in the form of a circle of radius 49 cm.
radius of circle r
= 49 cm
wire is in the form of a circle of radius 49 cm.
radius of circle r = 49 cm
side of square
radius of circle r = 49 cm = ?
= ?
side of square
Perimeter of the square = Perimeter of the circle
side of square = ?
Perimeter of the square = Perimeter of the circle
= 2πr
4a
Perimeter of the square = Perimeter of the circle
4a = 2πr
4a = 2πr 22
π 4a = 2 x 22 x 49

(or) = π d unit 2 4a 22 = 2 x 7 x 49
2
(or) π = 4 d unit 2 4a = 2 x x 49 7
2
(or) = 4 d unit 2 4 4a 7= 308
2
Circumference of the circle 2πr (or) πd unit
Circumference of the circle 2πr (or) πd unit 4a = 308
Examples
Circumference of the circle 2πr (or) πd unit 4a = 308 308
Examples a = 308
1 Find the area of a circle whose radius is 1.54 m. Also
Examples a 308 = 4
find its circumference.
1 Find the area of a circle whose radius is 1.54 m. Also a = 4
1 Find the area of a circle whose radius is 1.54 m. Also 4 = 77 cm
find its circumference.
radius r
find its circumference. = 1.54 cm = 77 cm
radius r = 1.54 cm 4 Find its radius if the difference between the
= 77 cm
= ?
radius r Area A = 1.54 cm 4 Find its radius if the difference between the
circumference and diameter of a circle is 28 cm.
= ?
Area A
Circumference C
4 Find its radius if the difference between the
circumference and diameter of a circle is 28 cm.
Area A = ? = ? 4 Find its radius if the difference between the circumference and diameter of a circle is 28 cm.
Circumference - Diameter = 28 cm
Circumference C = ? circumference and diameter of a circle is 28 cm.
A
2
Circumference C = ? = πr unit 2 Circumference - Diameter = 28 cm
2πr - d = 28
A = πr unit 2 Circumference - Diameter = 28 cm
2
2 22
A = πr unit = 22 x 1.54 x 1.54 2πr - d = 28
2
2πr - 2r = 28
22 = 7 x 1.54 x 1.54 2πr - d = 28
2πr - 2r = 28
7
= x 1.54 x 1.542 2r (π - 1)= 28
2πr - 2r = 28
= 7.4536 m
7 = 7.4536 m 2 2r (π - 1)= 28
C
= 2πr unit
= 7.4536 m 2 2r (π - 1)= 28
22
C = 2πr unit 2r ( 22 - 1) = 28
22
C = 2πr unit 22 x 1.54 22 7 - 1) = 28
2r (
= 2 x
7
22 = 2 x 7 x 1.54 2r ( - 1) = 28
x 1.54 7
= 2 x = 9.68 m 7 22 - 7
7 2r ( 22 - 7 ) = 28
= 9.68 m

7
2 Find out the circumference if the area of a circular shape 22 - 2r ( 7 ) = 28
= 9.68 m
of land is 616 m .
2 Find out the circumference if the area of a circular shape 2r ( ) = 28 7
2
2 Find out the circumference if the area of a circular shape 7 15
of land is 616 m .
2
A
= πr unit
2

2
of land is 616 m . A = πr unit 2 2r x 15 = 28
2
7

2
15
A = πr unit 2 616 2r x 2r x = 28 = 28

2
7
=
r
2

616
616 =
r 2 π 7 28x7
r 2 = π r = 28x7
π 616x7 r 15x2
28x7 =
= 616x7 r = 15x2
616x7 = 22 15x2 = 6.53 cm
22
= = 196 = 6.53 cm
22 55 = 6.53 cm
64 = 196 = 196 CITS : WCS - Electrical - Exercise 5
64
64