Page 71 - WCS - Electrical

5 What is the side of the largest square cut out from a
1
= x 50 x 16 cm
circle of 50 cm dia.?
2
2
Diagonal of a square = Diameter of the circle
= 400 cm
2
= 50
2a
r π
2
unit
Area of Semi circle
=
2
2
50
=
a
1
2
2
x
π
15
x cm
=
2
2
50
=
= 353.57 cm
2
1.414
Total area of the figure= 500 + 400 + 353.57
= 35.36 cm
= 1253.57 cm
2
6 Calculate the area of the figure given below.
steel plate 16 cm x 12 cm, there are 6 holes each 4
cm in diameter.
Area of a rectangular plate = length x breadth unit
2
= 16 x 12
= 192 cm
2
= 6
No. of holes
Radius of hole
= 2 cm
Area of rectangle
= lb unit
2
= 6 x πr unit
Area of 6 holes
2
2
= 25 x 20 cm
2
22
= 500 cm
x 2 x 2 unit
= 6 x
2
2
7
1
Area of Trapezium = x (a + b) h
= 75.43 cm
2
2
Area of remaining plate = 192 - 75.43
1
= x (30 + 20) 16 cm
2
= 116.57 cm
2
2
Semi circle
Examples
A semi circle is a sector whose central angle is 180º. 7 Find the area of remaining steel plate if in a rectangular
Length of arc of semi circle. 1 Calculate the circumference and area of a semi circle
whose radius is 6 cm.
180
= 6 cm
radius r
Length of arc  = 2πr x WORKSHOP CALCULATION & SCIENCE - CITS
360
Area A = ?
1
= 2πr x = πr unit Circumference c = ?
2
r π 2
r π 2 A = unit 2
Area of semi circle = Sq. units 2
2
22 1
= x x 6 2
7 2
22 1
Area (A) = x x 36
7 2
2πr 396
Perimeter of a semi circle = + 2r = = 56.57 cm 2
2 7
= πr + 2r 22
= r (π + 2) unit Perimeter of a semicircle = 6( 7 x 2)
⎞
WCS - Electrician & Wireman : (NSQF - Revised 2022) - 1 Year : Exercise 1.7.26 65
st

+ 14
⎛ 22
= 6⎜ ⎟
⎝ 7 ⎠
36
= 6 x
7
216
=
7
= 30.86 cm
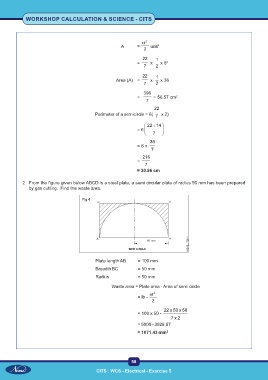
2 From the figure given below ABCD is a steel plate, a
Waste area = Plate area - Area of semi circle
2 From the figure given below ABCD is a steel plate, a semi circular plate of radius 50 mm has been prepared
⎞

semi circular plate of radius 50
+ 14
by gas cutting. Find the waste area. = 6⎜ ⎛ 22 mm has been prepared r π 2
⎟
by gas cutting. Find the waste area. = lb -
⎝ 7 ⎠ 2
Plate length AB = 100 mm
36
= 6 x
Breadth BC = 50 mm = 100 x 50 - 22 x 50 x 50
7
Radius = 50 mm 7 x 2
216
= 7 = 5000 - 3928.57
⎛ 22 + 14 ⎞ = 30.86 cm = 1071.43 mm 2
= 6⎜ ⎟
⎝ 7 ⎠
2 From the figure given below ABCD is a steel plate, a
36 Circular ring Waste area = Plate area - Area of semi circle
semi circular plate of radius 50 mm has been prepared
= 6 x 2 Find the distance between the boundaries and the area
r π
2
= lb -
7 by gas cutting. Find the waste area. of the circular ring, if the circumference of two concentric
2
216 Plate length AB = 100 mm circle are 134 cm and 90 cm.
= 22 x 50 x 50
7 Breadth BC = 50 mm Given: = 100 x 50 -
= 30.86 cm Radius = 50 mm Circumference of outer circle = 134 cm
7
2
x
= 5000 - 3928.57
2 From the figure given below ABCD is a steel plate, a Waste area = Plate area - Area of semi circle Circumference of inner circle = 90 cm
semi circular plate of radius 50 mm has been prepared 2 To find: = 1071.43 mm 2
by gas cutting. Find the waste area. R = Outer radius of circular ring r π Distance between the circles = ?
= lb -
Circular ring
Plate length AB = 100 mm r = Inner radius of circular ring 2 Area of circular ring = ?
2
2
Breadth BC = 50 mm Area of circular ring = π (R - r ) unit 2 22 x 50 x 50 2 Find the distance between the boundaries and the area
= 100 x 50 -
of the circular ring, if the circumference of two concentric
or Solution:
Radius = 50 mm A = π (R + r) (R - r) unit 2 7 x 2 circle are 134 cm and 90 cm.
= 5000 - 3928.57 Circumference of outer circle = 134 cm
1 Calculate the area of cross section of pipe having Given: 2 π R = 134 cm
= 1071.43 mm
2
outside dia of 17 cm and inside dia of 14 cm. Circumference of outer circle = 134 cm
Given: R =
Circular ring Outer dia of pipe = 17 cm Circumference of inner circle = 90 cm
2 Find the distance between the boundaries and the area
R = Outer radius of circular ring To find:
Circumference of inner circle = 90 cm
of the circular ring, if the circumference of two concentric
58
= 90 cm
2 π r
Outer radius of pipe (R) =
r = Inner radius of circular ring = 8.5 cm Distance between the circles = ?
circle are 134 cm and 90 cm.
Area of circular ring = π (R - r ) unit 2 Area of circular ring = ? =
Inner dia of pipe = 14 cm
CITS : WCS - Electrical - Exercise 5
2
2
Given:
r
or Solution:
Circumference of outer circle = 134 cm
Inner radius of pipe (r) =
A = π (R + r) (R - r) unit 2 = 7 cm Circumference of outer circle = 134 cm
Circumference of inner circle = 90 cm Distance between the circle = R - r
1 Calculate the area of cross section of pipe having
To find: 2 π R = 134 cm
= 21.32 - 14.32 cm
outside dia of 17 cm and inside dia of 14 cm.
To find:
R = Outer radius of circular ring Area of cross section of pipe = ?
Given:
=
r = Inner radius of circular ring Solution: Distance between the circles = ? R = 7 cm
Area of cross section of pipe = π (R + r) (R - r) unit 2 Area of circular ring = π (R + r) (R - r) unit 2
Area of circular ring = ?
Outer dia of pipe = 17 cm
Area of circular ring = π (R - r ) unit 2 Circumference of inner circle = 90 cm
2
2
or Solution: = π (8.5 + 7) (8.5 - 7) = π (21.32 + 14.32) (21.32 - 14.32) cm 2
2 π r
= 90 cm
A = π (R + r) (R - r) unit 2 Outer radius of pipe (R) = = 8.5 cm
Circumference of outer circle = 134 cm
1 Calculate the area of cross section of pipe having Inner dia of pipe = 14 cm = x 15.5 x 1.5 cm 2 = x 35.64 x 7 cm 2
= 134 cm
2 π R
r
=
outside dia of 17 cm and inside dia of 14 cm. 2
= 73 cm
Inner radius of pipe (r) = = 7 cm 2 = 784.08 cm
Given: R = Distance between the circle = R - r
To find:
Outer dia of pipe = 17 cm 66 Area of cross section of pipe = ? st = 21.32 - 14.32 cm
WCS - Electrician & Wireman : (NSQF - Revised 2022) - 1 Year : Exercise 1.7.26
Circumference of inner circle = 90 cm
Outer radius of pipe (R) = = 8.5 cm Solution: 2 π r = 90 cm = 7 cm
Area of cross section of pipe = π (R + r) (R - r) unit 2 Area of circular ring = π (R + r) (R - r) unit 2
Inner dia of pipe = 14 cm
=
r = π (8.5 + 7) (8.5 - 7) = π (21.32 + 14.32) (21.32 - 14.32) cm 2
Inner radius of pipe (r) = = 7 cm
Distance between the circle = R - r 2
To find: = x 15.5 x 1.5 cm 2 = x 35.64 x 7 cm
Area of cross section of pipe = ? = 73 cm 2 = 21.32 - 14.32 cm = 784.08 cm 2
Solution: = 7 cm
Area of cross section of pipe = π (R + r) (R - r) unit 66 Area of circular ring = π (R + r) (R - r) unit 2 st
2
WCS - Electrician & Wireman : (NSQF - Revised 2022) - 1 Year : Exercise 1.7.26
= π (8.5 + 7) (8.5 - 7) = π (21.32 + 14.32) (21.32 - 14.32) cm 2
= x 15.5 x 1.5 cm 2 = x 35.64 x 7 cm 2
= 73 cm 2 = 784.08 cm 2
st
66 WCS - Electrician & Wireman : (NSQF - Revised 2022) - 1 Year : Exercise 1.7.26