Page 46 - CITS - WCS - Mechanical

WORKSHOP CALCULATION - CITS

EXERCISE 8 : Statistics

Frequency tables

A frequency table consists of the lists of items in a given data set and the number of times each item occurs in the
data set. It is calculated by dividing the number of times a particular value for a variable has been observed, by
the total number of values in the population. For example, in a total of 20 coins tosses where are 14 heads and 6
tails, the proportion of heads is 0.7 (14 divided by 20).
The different types of frequency distributions are grouped frequency distributions, ungrouped frequency
distributions, relative frequency distributions and cumulative frequency distributions.
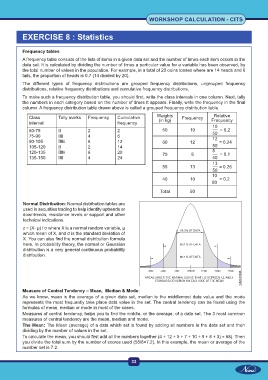
To make such a frequency distribution table, you should first, write the class intervals in one column. Next, tally
the numbers in each category based on the number of times it appears. Finally, write the frequency in the final
column. A frequency distribution table drawn above is called a grouped frequency distribution table.
Relative
Class Tally marks Frequency Cumulative Weights Frequency Frequency
(in kg)
interval frequency 10
60-75 II 2 2 50 10 = 0.2
50
75-90 IIII 4 6 12
90-105 IIIII 6 12 60 12 = 0.24
105-120 II 2 14 50
120-135 IIIII 6 20 70 5 5
= 0.1
135-150 IIII 4 24 50
13
55 13 = 0.26
50
10
40 10 = 0.2
50
Total 50

Normal Distribution: Normal distribution tables are
used in securities trading to help identify uptrends or
downtrends, resistance levels or support and other
technical indications.
z = (X- µ) / σ where X is a normal random variable, μ
which mean of X, and σ is the standard deviation of
X. You can also find the normal distribution formula
here. In probability theory, the normal or Gaussian
distribution is a very general continuous probability
distribution.

Measure of Central Tendency – Mean, Median & Mode:
As we know, mean is the average of a given data set, median is the middlemost data value and the mode
represents the most frequently take place data value in the set. The central tendency can be found using the
formulas of mean, median or mode in most of the cases.
Measures of central tendency, helps you to find the middle, or the average, of a data set. The 3 most common
measures of central tendency are the mean, median and mode.
The Mean: The Mean (average) of a data which set is found by adding all numbers in the data set and then
dividing by the number of values in the set.
To calculate the mean, you should first add all the numbers together (4 + 12 + 5 + 7 + 10 + 9 + 8 + 3) = 58). Then
you divide the total sum by the number of scores used (58/8=7.2). In this example, the mean or average of the
number set is 7.2.

33

CITS : WCS - Mechanical - Exercise 7